By Eng Carlos F Molina

Tank settlement was, for a long time, elusive to me. I got by only whith was needed for the exam. If you are an API 653 inspector, there are many opportunities to do inspection or consulting jobs in small tanks. The following is an introduction to the subject of settlement.

TYPES OF TANK SETTLEMENT

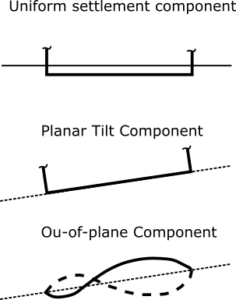
Settlement in tanks can be of several types. API 653 talks about three types: uniform, planar tilt and out-of-plane settlement.

[adToAppearHere]

Uniform settlement. This component often can be predicted in advance, with sufficient accuracy, from soil tests. Uniform settlement does not induce stresses in the tank structure. However, attention should be paid to pipe connections and other attachments.
Rigid body tilting of a tank (planar tilt). This component rotates the tank in a tilted plane. The tilt will cause an increase in the liquid level and, therefore, an increase in the hoop stress in the tank shell. Failures of most concern are: (1) Distortion of shell such that the floating roof malfunctions; and (2) rupture of the shell or bottom plate or shell bottom plate connection. The settled location of the tank shell, after rigid body tilt, can be represented by either a cosine or sine wave with respect to its original position in a horizontal plane.
Out-of-plan settlement.While uniform settlement and rigid body tilt of a tank may cause problems, the out-of-plane settlement is the important component to determine and evaluate in order to ensure the structural integrity of the shell and bottom. Based on this principle, a common approach is to determine the magnitudes of the uniform settlement and rigid body tilt (if a rigid tilt plane exists or can be identified) for each data point on the tank periphery.

THE COSINE CURVE

For the study of settlement (general settlement of the shell) let´s establish a common notation.

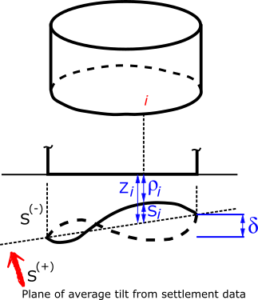
D = diameter of tank
H = height of tank
S = out-of-plane settlement
Si = out-of-plane settlement of point i = component of settlement of point i due to out-of-plane-distortion
Rho =  measured settlement of point i
delta  = difference in settlement between diametrical points
Zi = component of settlement of point i due to planar tilt

We will use this notation later. Not in this article.

The plane of rigid tilt settlement, if well-defined, is represented by the optimum cosine curve. Several methods exist for determining the optimum cosine curve. The least accurate method is by free hand drawing techniques, a kind of trial and error procedure to fit the best cosine curve through the data. A better method is to use the mathematical and graphical capabilities of a computer

This cosine curve is acceptable if the value R2 is greater than or equal 0.9

HOW TO FIND THE COSINE CURVE

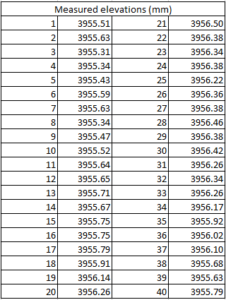
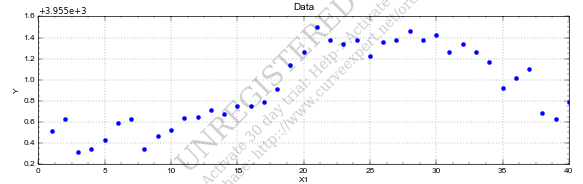
Let´s suppose we have the following set of data of a tank.

N=40

Procedure

1. Feed the measured settlement data to a curve fitting software. In this case we will use Curveexpert professional, the trial version.

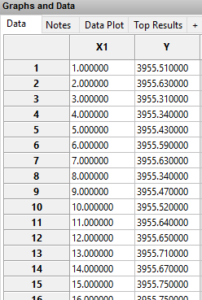
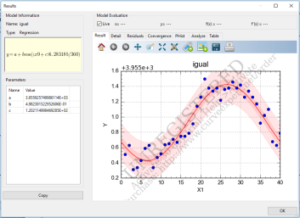
When you feed the data, it builds the point graph with the measured data

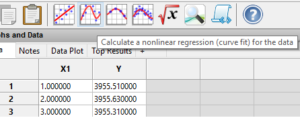
2. Choose the type of curve. Click non-linear regression.

3. Feed the software with your custom correct equation, based in the one from API 653. I called my equation “igual”, and it the following

The 9 stands for the 9 degrees between each measurement point. The rest stands for the deg/rad conversion.

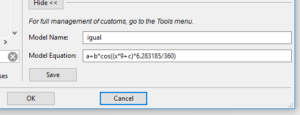
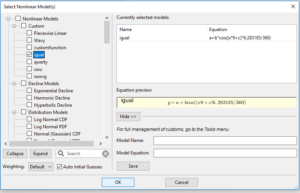
Click Ok. The software will give you values for constants a, b and c

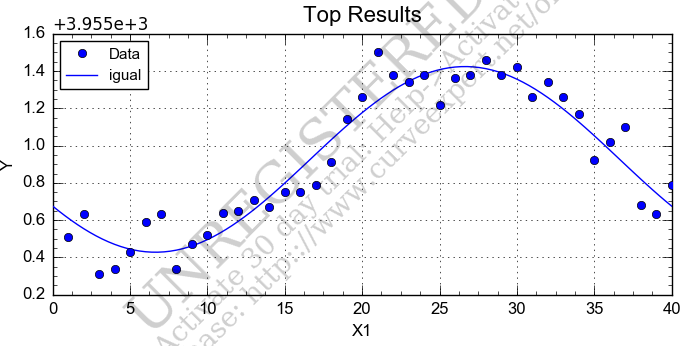
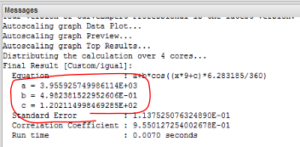
In this example.

a=3955.92
b=.498
c=120.2 degrees

[adToAppearHere]

4. Make sure that the “Graphs and data” and “Results” windows are open

5. In the “detail” tab, you can find the value of R2

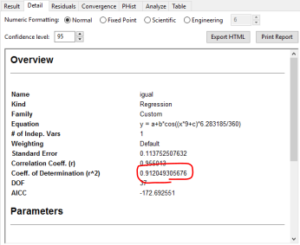
R2 =0 .912  OK

Besides, curveexpert, I used Microsoft Excel to find the value of R2. With excel, I found a value of R2=0.888. This is because I used 40 points, while Curveexpert uses I don´t know how many more. Lesson is: the software is better approximating a curve.

The cosine curve in this example fullfills the requirement of API 653. But what happens when it doesn´t fulfill the requirements? When there is no optimal cosine curve? That will be the subject matter of another article.

Thanks for reading.